What is Big Seven strategy for division?

The Big 7 division method is a useful way to introduce long division using partial quotients. By partial quotient, we refer to the factor or number that we multiply the divisor by.

I often use this partial quotient method to introduce students to division.

The name comes from the fact that the division ‘house’ is extended to look like a big seven. Students solve the long division problem by subtracting easy multiples of the divisor, for example, 10x, 5x or 2x at a time until they reach 0 or as close to 0 as possible. These partial quotients are recorded on the outside of the Big 7 with the subtractions shown on the inside of the Big 7.

Students can find different partial quotients for the same problem but I try not to encourage subtracting multiples of 1 every single time! It is quite fun to show students why this is not the best way with a very long length of paper!

Setting up the Big 7 Division Problem

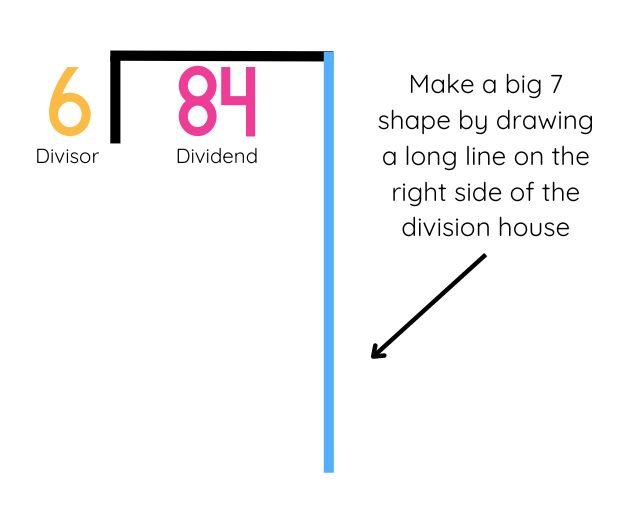
In order for you to teach this method to your students, let’s work through an example, 64 ÷ 6.

In this first step, draw a division ‘house’ with the dividend (64) inside the ‘house’ and the divisor (6) outside of the ‘house’. Make a big 7 by drawing a long line (shown in blue) on the right side of the division house as shown in the diagram below.

Now let’s go through the process of solving the problem step by step. Remember this is one way of calculating the answer, students can use other partial quotients to the ones used below.

STEP 1

First, et’s determine how many sets of 6 can fit into 84. Use ‘easy’ multiples like x10 or x2 to make calculations easier.

Recognizing that at least 10 sets of 6 fit into 84, we begin with that as out first subtraction from 84.

We denote this by writing 10 on the right side of the big 7, indicating the removal of 10 sets. This is our first partial quotient.

We are going to subtract these 10 groups of 6, totalling 60 from the dividend. The total, 60, is written under the dividend 84 as shown below.

Step 2

Subtract 60 from 84 and write the answer, 24, underneath.

STEP 3

Subtracting 60 from 84 has left us with 24. We now need to work out how many groups of 6 are in 24.

In this example I’m going to use an easy multiple of x2 so 2 x 6 = 12. You can see that I have written the number 2, which represents the number of sets I’ve removed (the partial quotient) on the right side of the seven again. The total 12 is subtracted from 24.

Step 4

I’m going to repeat this process one more time. Subtracting 12 from 24 has left us with 12. We can subtract another 2 sets of 6, recording the partial quotient (2) on the right of the big 7 and the total 12 on the left side under the previous calculation as shown below:

STEP 5

12-12 = 0 which means we have subtracted all possible groups of 6. We will now add up all the partial quotients which in this case is 10 + 2 + 2 = 14. This gives us the answer to our problem:

84 ÷ 6 = 14.

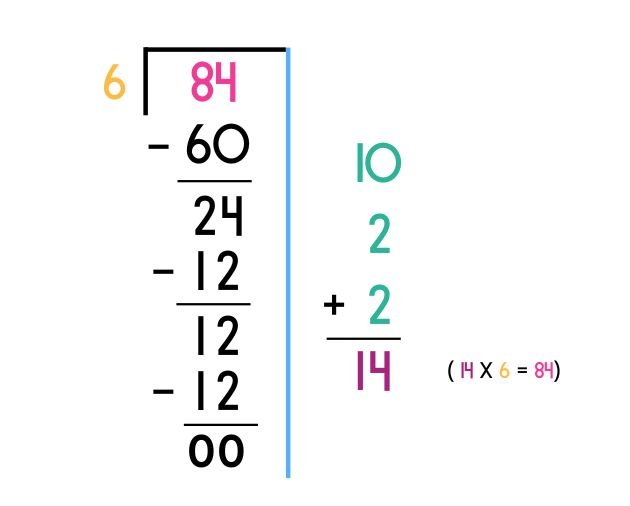
A few quick tips!

Consider letting the students work in pairs to start off with. Whilst one completes a question, the other students act as a guide, explaining the process, then switch over. I find this helps students begin to understand what is needed and commit the steps to memory better.

Students will need a good understanding of multiplication facts/times tables, in particular x10, x5 and x2. It’s worth doing starter activities that help students practice these before starting your lesson on partial quotients long division.

It can be helpful to have multiplication charts in front of your students on their desks and also a mini anchor chart for those who need it.

Start with smaller numbers and build up, for example, begin with 2 digit by 1 digit divisions to help students grasp the concept. As understanding grows, I gradually increase the difficulty to include 3 digit and 4 digit numbers.

If you’d like to know more about other methods of teaching long division, please follow these links to read:

The Box Method Division Method or How to teach long division to upper elementary

Resources to support your teaching of long division

Jumping Elephant Math long division resources are designed to meet the needs of a variety of learners and align with common core standards. Check out these bestselling long division resources in my store.

I’d love to hear how you found using this method with your students, so please send me an email with any comments to [email protected]. I look forward to hearing from you!